Low distortion cathode / emitter /source follower
Many believe that a cathode follower (or emitter, or source follower) has very low distortion due to a 100% negative feedback. But in fact a conventional follower may have a substantial distortion. It depends on the type of an active device, bias, load, signal strength and signal source impedance. Consider a generic follower:
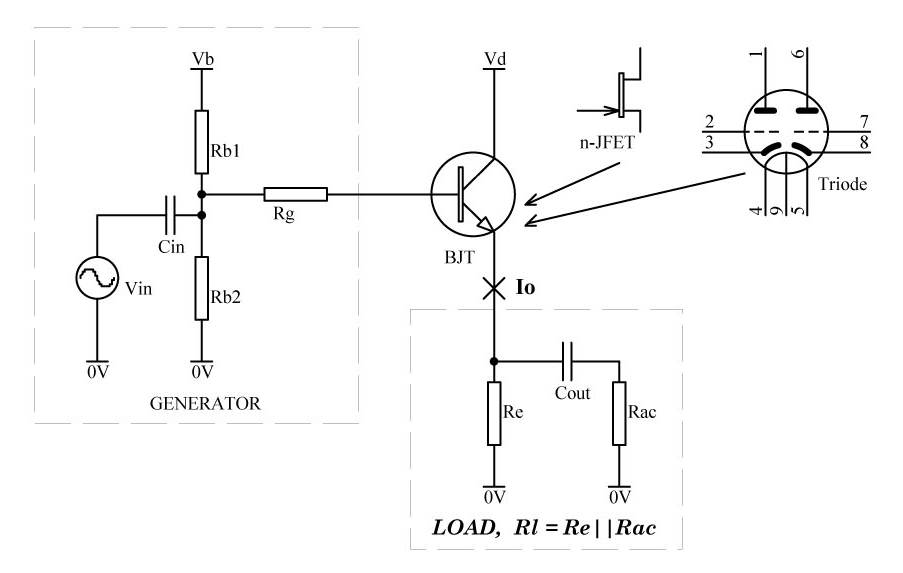
Fig. 1
Vin -- input signal amplitude, V;
Vd -- voltage across cathode-plate, emitter-collector or source-drain, V;
RL -- AC load resistance, parallel of the cathode bias resistor and AC load resistance, kOhm;
Rg -- signal source resistance, kOhm;
I0 -- quiescent bias current (DC component), mA;
S -- transconductance of an active device, where applicable, mA/V;
µ -- amplification factor of an active device, where applicable;
B -- common emitter current gain of a bipolar transistor, "beta";
D -- distortion (nonlinearity), ratio, not %.
The following crude formulae can be used to assess nonlinearity (2-nd harmonic only) of a follower using different active devices: a bipolar transistor (BJT), a field-effect transistor (FET), a vacuum tube (triode).
1. Bipolar transistor
For an emitter follower:
D = ½ * (0.025V / (RL * I0) * (Vin / ( RL * I0) + (Rg / RL) * ((0.05...0.2) / B) * (Vin / ( RL * I0) (1)
The first term gives distortion related to the emitter junction nonlinearity, the second term -- distortion caused by beta variations with the changes of collector current. Magnitude of it depends on the transistor type, therefore some range 0.05...0.2 is quoted. 0.025V is a thermal voltage of a transistor.
For example, with I0 = 1mA, Vin = 1V, RL = 2K emitter nonlinearity gives 0.3% of distortion (first term of the equation). With Rg = 10K and beta B = 200, another 0.05...0.25% of distortion is added on top of that (from the second term of the equation). Distortion is not that low after all.
2. Field effect transistor
For a source follower:
D = ¼ * (1 / (S * RL)) * (Vin / ( RL * I0)) + (0.1...0.5) * (Vin / Vd2) (2)
Again, the first term evaluates gate control nonlinearity (quadratic function), the second term -- nonlinear dependence of current on the drain-source voltage which changes with the signal.
For example, with I0 = 1mA, Vin = 1V, RL = 2K, S = 3mA/V transconductance nonlinearity will result in about 3% of distortion, and with drain-source voltage Vd = 10V one can expect another 0.1...0.5% from the drain characteristic curvature. In fact, for a resistive this component of distortion will likely subtract from the first term, but in general, to be on the conservative side, for a reactive load, it is to be added. We can see that the source follower performance at low bias currents is even worse than of a BJT.
3. Vacuum tube (triode)
For a cathode follower:
D = (1/6) * (1 / (S * RL)) * (Vin / ( RL * I0)) +
(0.005...0.3) / µ) * (Vin / ( RL * I0) +
(0.005...0.3) / µ) * (Vin / Vd) (3)
The first term evaluates control grid nonlinearity (law of 3/2), the second term -- nonlinear dependence of the amplification factor µ on the cathode-plate voltage which changes with the signal. The first term is similar to the source follower case, but the factor in front is 1/6 compared to 1/4 for a source follower. This is because a valve is inherently more linear -- plate current is proportional to (voltage)3/2 as opposed to (voltage)2 for a FET. Nevertheless, because of relatively low transconductance, a cathode follower performs poorly at low currents. With I0 = 1mA, Vin = 1V, RL = 2K, S = 1mA/V it will have about 4% of distortion. Besides, the gain of such follower is quite low -- only about 0.66. The second term accounts for µ variation due to changing plate current, while the third term -- for µ variation due to plate-cathode voltage changing. It is difficult and unnecessary to precisely analytically describe the interplay of these effects. At a certain plate voltage and plate current some triodes might exhibit very stable, nearly constant µ, thus making this distortion mechanism negligible. That is why the range of coefficients 0.005...0.3 is so wide.
Load resistance RL has the most profound (quadratic) effect on distortion figures. It should be increased as much as possible by increasing Re if possible or using a constant current source. Idle current I0 also needs to be as high as practical. Such measures result in circuit complexity and still do not drastically reduce distortion of a cathode follower.
Is there a way to radically reduce distortion of a cathode follower? The answer is: "Yes".
4. Boosted compound cathode follower
A solution is quite simple -- to replace Re with an active common emitter transistor and apply feedback. The simplest solution is depicted in Fig. 2.

Fig. 2
In the improved cathode follower (Fig. 2) the tube V1 no longer supplies AC output current. Instead the valve works only as an error amplifier, comparing the difference between input (grid) and output (cathode). Voltage difference is converted into plate current by the transconductance of the tube, and this error current applies to the base of Q1 booster BJT which in fact drives the output. Here B1 is the current gain (beta) of the booster transistor Q1.
Thus, AC current through the tube is B1 times less than in a conventional follower (Fig. 1). The tube (or any other type of an active follower device) virtually works with almost constant plate current.
Immediate effects of the boosted follower topology are obvious:
- Output resistance drops B1 times -- typically below 5 Ohm;
- Input impedance increases B1 times (especially useful for an emitter follower);
- Gain of the follower increases coming closer to a theoretical limit: for a tube -- about µ / (µ+1); for BJT -- typically 0.999; for a FET -- 0.95...0.999, depending on the supply voltage Vd.
Distortion is reduced, but its components are different.
D = (1 / (2*µ)) * (0.025V / (RL * I0) * (Vin / ( RL * I0) +
+ (1 / (S * RL)) * ((0.05...0.2) / B1) * (Vin / ( RL * I0) +
+ ((0.005...0.3) / µ) * (Vin / Vd) (4)
Control grid nonlinearity (first term in (3)) is reduced B12 times, making this component negligible. This term can be omitted.
The first term of (4) represents Q1 voltage distortion, reduced µ times because of the triode. With µ = 50, I0 = 1mA, Vin = 1V, RL = 2K this gives about 0.006%.
The second term evaluates B1 nonlinearity. If B1 = 200 and S = 1mA/V, it will give 0.006...0.03%. It depends on how stable B1 is when collector current swings.
The third term in (4) relates to µ being not constant. But in this case, since the plate current is almost constant, only input plate-cathode voltage can affect µ. Therefore only the third term from (3) applies to the boosted cathode follower. This term is exactly as in (3) and is not reduced by the booster transistor Q1. Under the above conditions, with Vin = 1V and Vd = 150V, it appears negligible: 0.0001...0.004%, but might rise if the cathode follower is intended for higher input voltages. It is "smooth" with mostly the second and third harmonics. Nevertheless, it is advisable to choose voltage and current through the tube V1 which yields the most "flat" µ.
For a source follower an equation similar to (4) will apply, but the third "µ-term" will dominate, and it is difficult to reduce it unless a high voltage MOSFET and high supply voltage Vd are used. So source boosted followers are not recommended.
The most dramatic effect can be achieved by boosting an emitter follower. Since effective µ of a BJT is very high -- 1000...20000, only the second (middle) term will be of any significance, yet about 10...40 times smaller than that of a tube because transconductance of a BJT is higher than of a valve for the same current.
A major drawback of the circuit in Fig. 2 is that R4 might need selection test, depending on B1 variation, to obtain a desired plate voltage for the tube V1. Circuits in Fig. 3 and Fig. 4 are free from such inconvenient drawback.
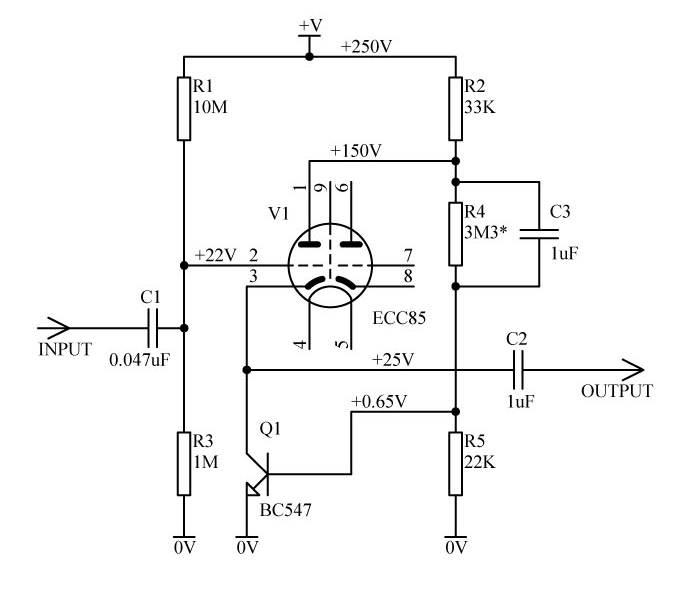
Fig.3
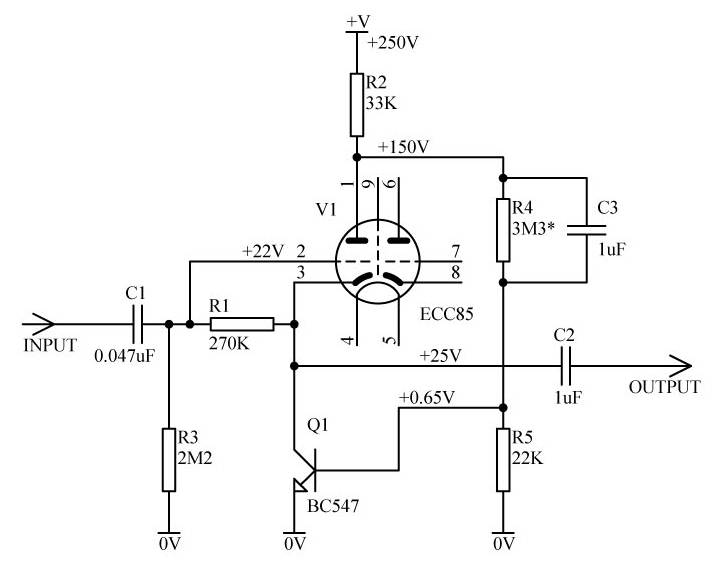
Fig. 4
In these circuits, plate voltage equals approximately 0.65V * (R4 / R5) and depends little on B1. To handle large signals, positive grid bias can be applied through a fixed divider R1R3 (Fig. 3) or through a "self bias" divider (Fig. 4). The later is more sensitive to tube parameters, but does not feed power supply noise to the input and does not stress the tube with positive grid voltage on warm-up. Grid stoppers (not shown) are highly recommended. Such followers work well even into capacitive loads, however, if in rare cases oscillation happens, a small capacitor (several pF) can be connected across the collector junction of Q1. In order not to reduce loop gain, equivalent resistance of the paralleled R2 and R5 should be larger than input resistance of the booster transistor Q1:
R2||R5 > (0.025V / I0) * B1 (5)
Usually this condition (5) is not difficult to satisfy.
This compound boosted cathode follower topology is somewhat similar to the so called White cathode (emitter) follower (Fig. 5), but there are differences.
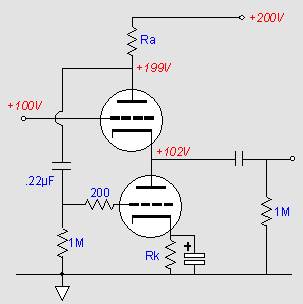
Fig. 5. White cathode follower.
In the White follower both the follower and the booster active devices (tubes) are identical and R2 is reciprocal to the transconductance of a booster device: Ra = 1 / S. Due to low Ra, loop gain around both triodes is only 1. The aim of the White follower is to double the output signal amplitude, like in a push-pull class A amplifier. Both triodes share the load. Output current amplitude of the White follower is twice its quiescent current I0. Output impedance reduces in half, and even harmonics are compensated to some extent. Other harmonics are reduced as well, but not significantly.
In contrast to that, in the compound boosted cathode follower (Fig. 2 - 4) the top triode does not contribute to driving the load at all. Only the bottom booster transistor drives the load. Output current amplitude can reach only I0 , like in a single-ended class A amplifier. Loop gain is high, about B1 , which drastically reduces output resistance and harmonics, except for the component caused by "non-flatness" of µ.
5. Variations of amplification factor µ of a triode
Stable amplification factor µ is essential for an extra-low distortion boosted cathode follower. µ is already the most invariable parameter of a triode, yet it is not perfectly constant. µ is associated with the level of protection, shielding, screening of the cathode by the control grid from the electrostatic field of the plate. The higher the protection -- the greater the µ.
A triode can be considered as a parallel connection of infinitely small triodes along the length of the cathode. Those sections of the cathode right under the grid wires have the best protection from the plate field. Those sections right between the grid wires are the most exposed to the plate field or the less protected.
Consider a triode biased close to cut-off. In this case only the electrons emitted from the small sections of the cathode between the grid wires are capable to "squeeze" as a narrow sheet beam right in the middle between the grid wires. All other sections of the cathode are below cut-off -- not contributing to valve operation. Thus only the portions of the triode with lowest µ are in operation close to cut-off. The overall µ is therefore low.
Now consider the triode running at a small negative bias. Less negative bias now allows the emission from almost the whole stretch of the cathode to contribute to the plate current. "Vulnerable", exposed low-µ sections between the grid wires work together with more protected areas under the grid wires. Thus low-µ sections work in parallel with high-µ sections, and the overall average µ becomes higher.
Similarly, µ depends on the bias because of not completely planar and not ideally concentric construction of a triode. Close to cut-off only those sections closest to the plate, the most vulnerable, work. At mild bias, all the plate works resulting in µ increase.
If the grid bias rises further into zero or positive range, then the grid draws large numbers of the electrons from the whole cathode area regardless of the plate voltage. This space charge expands to the grid. Virtual cathode moves from the physical surface of the cathode closer to the grid, thus becoming more vulnerable. Hence µ goes down again.
Thus a triode has a maximum of µ at a certain bias and plate voltage. Operation point where µ is the highest corresponds to the minimum distortion of the boosted cathode follower. Peak µ point depends on the type and construction of a triode. A triode with the flattest µ needs to have a concentric construction with the fine grid away from the cathode. Then field at the cathode would be uniform. Effects of each individual grid wire would be smoothed and averaged. (It is like standing on a sunny day under a tall tree, where you can not see projections of each leaf and branch on the ground, but experience a uniform semi-shade.) Such a triode would have a very low transconductance and not useful for general purpose. Therefore such special triodes for the µ-followers have not been manufactured. However, beam tetrodes controlled by the screen grid exhibit good linearity of µ. It is because the screen grid is farther from the cathode.
Even different makes of a tube with the same part number can have different peak µ points. In most tubes, maximum µ can be achieved only at close to zero or even positive grid bias, which is impractical. In some tubes, maximum µ can be obtained at a modest negative bias. Such tubes are suitable for the extra low distortion cathode follower.
Maximum µ point can be found experimentally by achieving minimum distortion of an unloaded cathode follower at high signal level. It is not easy as the distortion is very low from the start. In practice it is easier to tweak for the maximum, closest to unity, gain. Changing R4 and R5, one can adjust plate voltage, varying R2 -- plate current (Fig. 2 - 4).
6. Boosted emitter follower as a power audio amplifier
Booster emitter follower topology can be used to build a low power audio class A amplifier-follower, to drive headphones or a small speaker. A sample circuit is shown below.

Fig. 6. Low power audio emitter follower
Here Q1Q2Q3 is the composite emitter follower with very high current gain (product of the three betas) and therefore high input resistance. Q7Q6 is the composite booster stage. Its equivalent beta B1 reaches about 10000. For that reason output resistance of this follower is in micro-ohm range, gain is 0.999 or higher, distortion is almost impossible to measure. Actual output impedance and distortion are determined by the output capacitor C5.
Instead of a current supplying resistor R2 (as in Fig. 3 and 4), a constant current source Q4Q5 is used. It allows to minimise voltage loss, and yet have very high dynamic impedance to easily satisfy condition (5).
In this sample circuit, idle current is about 650mA. Transistors Q3, Q6 and Q4 need to be appropriately rated and mounted on the heatsinks. DC stabilisation via p-JFET Q8 maintains some voltage drop, close to the cut-off voltage of Q8, across Q4. Capacitor C2 is optional and needed only if parasitic oscillation occurs. Also, parasitic oscillation might develop in the composite transistor Q1Q2Q3. If this happens, one of the collector junctions should be shunted by a small capacitor. A reverse parallel diode is recommended across Q7 emitter junction to prevent reverse biasing on overload or power-down.
